The Moscow State Institute of Radio Engineering, Electronics and Automatics (Technical University). E-book (DjVu-file) contains solutions of 7 typical problems for the first-year students of full-time education.
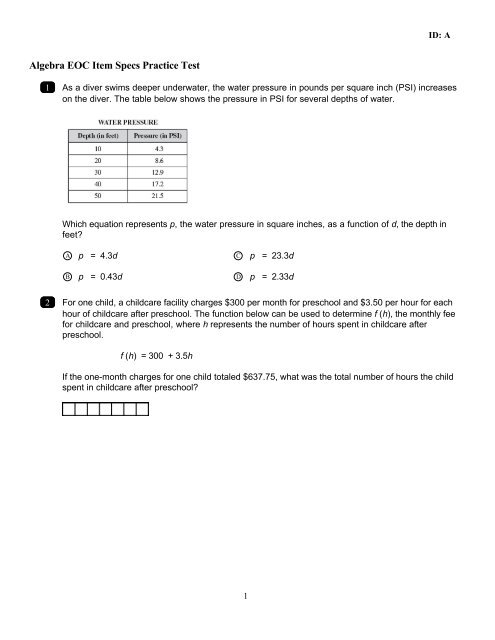
Sbornik Zadach Po Linejnoj Algebre. Puzzles dover, moral foundation state hegels philosophy, moodle 1.9 testing assessment myrick, moon magic. Results 1 - 30 of 66 - Lektsii po matematike. Linejnaya algebra. Boss and a great selection of related books, art and collectibles available now at.
Problems are taken from the from the task book in Algebra and Geometry developed for MIREA students. Authors: I.V.Artamkin, S.V.Kostin, L.P.Romaskevich, A.I.Sazonov, A.L.Shelepin. Izotope nectar elements v1001047 download free software. Einsam zweisam dreisam film download. Yu.I.Hudak Editor (Publisher MIREA 2010).
Problem solutions are presented in the form of scanned handwriting papers collected into a single document of 14 pages. This document is saved in the DjVu-format which can be opened in the Internet Explorer or Mozilla Firefox browsers with the aid of the DjVu plug-in. Links to download and to install DjVu plug-in are attached. DjVu-file containing the problems and their detailed solutions is ready for viewing on a computer and for printing. All solutions were successfully accepted by MIREA teachers.
Problems of the Typical calculation: Problem 1. The surface of the second order σ is given by its equation in a rectangular Cartesian coordinate system. 1) Determine the type of the surface σ. 2) Draw the surface σ.
3) Draw cross-sectional surfaces of the surface σ by coordinate planes. Find the foci and asymptotes of the obtained curves. 4) Determine, on one or on opposite sides of the surface σ do the points M1 and M2 lie.

5) Determine how many points of intersection with the surface σ has a straight line passing through the points M1 and M2. Given a complex number z. 1) Write down the number z in the exponential, trigonometric and algebraic forms and display it in the complex plane.
2) Write in the exponential, trigonometric and algebraic forms the complex number u=z^n, where n=(-1)^N*(N+3) for N≤15, n =(-1)^N*(N-12) for N≥16, N - number of variant. 3) Write the exponential and trigonometric forms for the roots of m-th degree of z: w_k (k = 0, 1., m - 1) m = 3 (odd variants), m = 4 (even variants).
4) Display the number z and the numbers w_k on one of the same complex plane. Given a polynomial p(z)=a*z^4+b*z^3+c*z^2+d*z+e. 1) Find the roots of the polynomial p(z). Write each root in the algebraic form and specify its algebraic multiplicity. 2) Arrange the polynomial p(z) into irreducible factors: a) a set of complex numbers - C; b) a set of real numbers - R.
Let P_n - linear space of polynomials of degree at most n with real coefficients. The set M from P_n consists of all polynomials p(t), which satisfy the above conditions. 1) Prove that M - subspace P_n.
2) Find the dimension and a basis for the subspace M. 3) add to the basis of the subspace M a basis for P_n. Prove that the set M forms a subspace of mxn matricies of a given size.
Find the dimension and a basis for a set M. Check that the matrix B belongs to the set M and find its coordinates in the M basis.
Prove that the set of functions M x(t), defined on the domain D is a linear space. Find its dimension and basis. Given the vectors a=OA, b=OB, c=OC, d=OD. The rays OA, OB and OC are the edges of trihedral angle T. 1) Prove that the vectors a, b, c are linearly independent. 2) Express the vector d via the vectors a, b, c (solve the related linear system of equations with the aid of inverse matrix). 3) Determine whether a point D is inside the T or D is on one of the boundaries of T (on what?).
4) Determine for which values of real parameter λ vector d + λa, with the begining in the point O, lies inside the trihedral angle T.
Posts
- Macfamilytree 6 Keygen Torrent
- Spiderman 3 Pc Game Sound Files
- 3dsimed Keygen Crack
- Magic Engine 113 Key
- Francaix Clarinet Concerto Pdf
- Download Free Force Op Minecraft152 Newexploit
- Programmu Proverki Znanij Ekzamen Versiya 158
- Vietcong No Cd Patch 16
- Prezentaciya Na Temu Ekonomika Ssha Na Anglijskom Yazike
- Cabela Dangerous Hunts 2009 Ps2 Iso Torrent
- Illegal Tender Soundtrack Songs Mp3 Download
- Fate Hollow Ataraxia Save Game
- Flash Card Belajar Membaca Huruf Hijaiyah
- Free Download Game Pc Strategi Perang Kerajaan Tiongkok